Understanding the Probability of Four Options: A Comprehensive Guide

Calculating the Probability of 4 Options

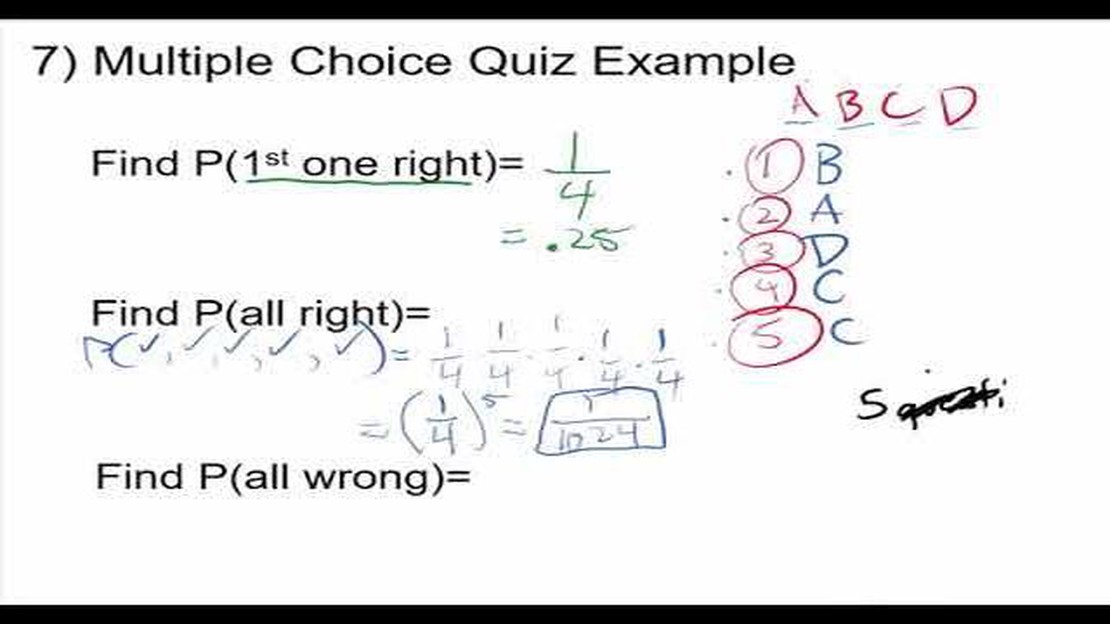
Have you ever wondered about the probability of four different options? Whether you’re a statistics enthusiast or just curious about the odds, this comprehensive guide will delve into the intricacies of probability and provide you with a clear understanding of the subject.

Probability is a fundamental concept that plays a crucial role in various fields, from mathematics and economics to science and everyday decision-making. In this guide, we will focus specifically on the probability of four options and explore how it can be calculated and analyzed.

Table Of Contents

First, we will define what probability is and how it is measured. Probability is essentially a measure of the likelihood that a specific event will occur, expressed as a number between 0 and 1, where 0 represents impossibility and 1 represents certainty. Understanding this basic concept is essential for comprehending the probability of four options.

Next, we will discuss different mathematical approaches to calculating probabilities, such as the classical, empirical, and subjective methods. Each method has its own merits and can be applied in different scenarios. By understanding these methods, you will be better equipped to analyze the probability of four options accurately.

Furthermore, we will explore real-world applications of probability, from business and finance to sports and gambling. Through practical examples and case studies, we will demonstrate how probability is used to make informed decisions and assess risks in these fields.

By the end of this comprehensive guide, you will have a solid foundation in understanding the probability of four options. Whether you want to enhance your mathematical skills or simply gain a deeper appreciation for probability, this guide will provide you with the knowledge and insights you need.

The Basics of Independent Events

Understanding the probability of four options requires a solid grasp of the basics of independent events. Independent events are events in which the outcome of one event does not affect the outcome of another event.

When dealing with independent events, it’s important to remember that the probability of each event occurring remains constant throughout the process. This means that the probability of one event occurring does not impact the probability of any subsequent events.

To calculate the probability of independent events occurring together, you can simply multiply the probabilities of each individual event. This is known as the multiplication rule of probability. For example, if the probability of event A occurring is 0.3 and the probability of event B occurring is 0.5, then the probability of both events occurring together is 0.3 * 0.5 = 0.15.

It’s also important to note that the multiplication rule of probability can be applied to any number of independent events. If you have three independent events with probabilities of 0.4, 0.6, and 0.2, respectively, you can find the probability of all three events occurring together by multiplying the probabilities: 0.4 * 0.6 * 0.2 = 0.048.

When working with independent events, it’s essential to keep in mind that past events do not influence future events. Each event should be treated as a separate and unrelated occurrence.

Read Also: Discover the Czech Republic's Largest Trading Partner | Uncover the Key Economic Relationship

In conclusion, understanding the basics of independent events is crucial when calculating the probability of four options. By recognizing that the outcome of one event does not impact the outcome of another, and applying the multiplication rule of probability, you can accurately determine the probability of multiple independent events occurring together.

Calculating Probability: The Law of Large Numbers

In probability theory, the law of large numbers is a fundamental concept used to understand the long-term behavior of random events. It states that as the number of trials or observations increases, the experimental probability of an event will converge to its theoretical probability.

Read Also: Understanding the Stochastic RSI with a 14 14 3 3 Setting: A Comprehensive Guide

This law provides a way to estimate probabilities using statistics and data analysis. By collecting a large enough sample size, we can make reasonable predictions about the likelihood of an event occurring.

For example, let’s consider a fair six-sided die. The theoretical probability of rolling a specific number, such as a 4, is 1/6, or approximately 16.67%. Now, if we were to roll the die 100 times, we would expect to see the number 4 approximately 16.67% of the time. However, it is possible that in those 100 rolls, we may see the number 4 more or less than 16.67% of the time. This is where the law of large numbers comes into play.

As we increase the number of rolls, say to 1000 or 10,000, the experimental probability of rolling a 4 will start to converge to its theoretical probability. This means that the more trials we conduct, the closer our observations will align with the expected probability.

The law of large numbers is essential in many fields, including finance, gambling, and insurance. It helps professionals make informed decisions by understanding the likelihood of certain events occurring based on past data and observed probabilities.

It is worth noting that the law of large numbers does not guarantee that the experimental probability will always align perfectly with the theoretical probability. There can still be some variation due to random chance or other factors. However, as we increase the sample size, the difference between the two probabilities becomes smaller and more predictable.

In conclusion, the law of large numbers is a powerful concept that allows us to calculate probabilities by analyzing large sets of data. It helps us make informed decisions and predictions, providing a deeper understanding of the likelihood of specific events occurring.

FAQ:

What is the purpose of this guide?

The purpose of this guide is to provide a comprehensive understanding of the probability of four options and how to calculate it.

How can I calculate the probability of four options?

To calculate the probability of four options, you need to use the formula for calculating the probability of an event, which is the number of desired outcomes divided by the total number of possible outcomes.

Can you give an example of calculating the probability of four options?

Sure! Let’s say you have a bag with 10 marbles: 3 red, 2 blue, 2 green, and 3 yellow. To calculate the probability of picking a red marble, you divide the number of desired outcomes (3) by the total number of possible outcomes (10), giving you a probability of 3/10.

Are there any specific techniques or strategies to improve understanding of probability?

Absolutely! One technique is to practice with various probability problems, such as rolling dice or drawing cards. Another strategy is to break down complex probability problems into smaller, more manageable steps. Additionally, learning about probability concepts, such as independence and conditional probability, can help improve understanding.