Understanding the Normal SABR Model: A Comprehensive Guide

The Normal SABR Model: An Introduction to Stochastic Volatility Modeling

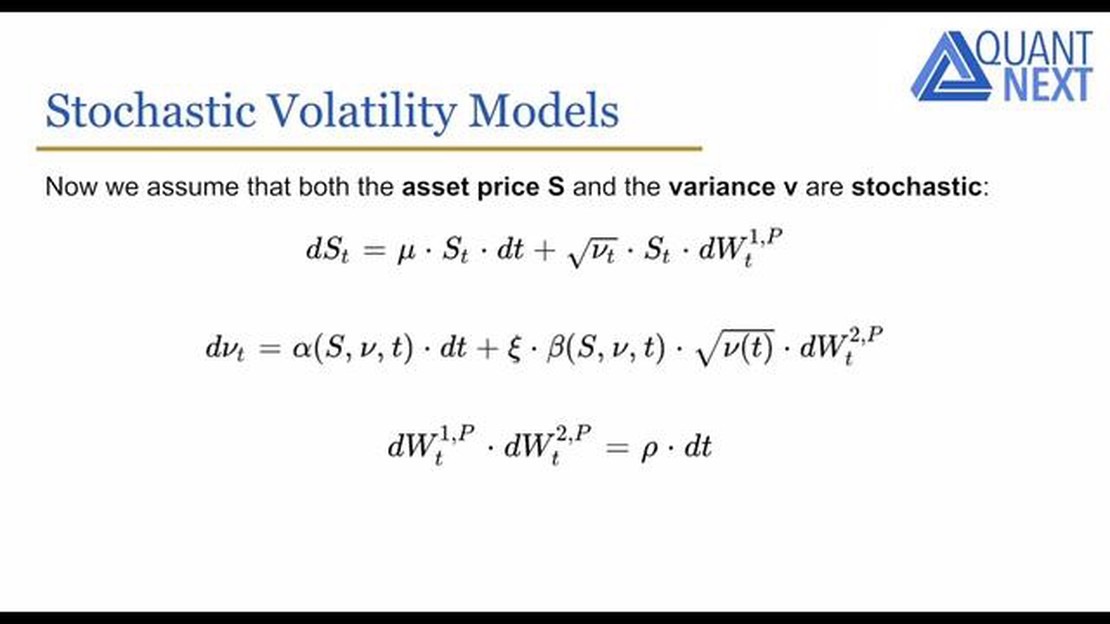
The Normal SABR (Stochastic Alpha Beta Rho) model is a widely used mathematical framework for pricing and hedging volatility derivatives. It was first introduced by Hagan et al. in 2002 as an extension of the original SABR model, providing a more flexible and realistic representation of option prices. In this comprehensive guide, we will delve into the intricacies of the Normal SABR model and explore its various applications in quantitative finance.

Table Of Contents

The Normal SABR model considers the dynamics of a financial asset’s volatility, taking into account three key factors: the asset’s price, the time to expiration, and the volatility itself. It assumes that the volatility follows a normal distribution, which is a reasonable approximation for many financial assets. This assumption allows for efficient pricing and calibration techniques, making the Normal SABR model a popular choice in the industry.

One of the main advantages of the Normal SABR model is its ability to capture the volatility smile, a phenomenon commonly observed in option markets where at-the-money options have a lower implied volatility compared to out-of-the-money options. By incorporating a skew parameter, the Normal SABR model can accurately reproduce this market behavior and provide more accurate option prices.

In addition to pricing options, the Normal SABR model has various applications in risk management and trading strategies. It can be used to calculate Greeks, such as delta and gamma, which indicate the sensitivity of an option’s price to changes in the underlying asset. These Greeks are crucial for managing portfolio risk and optimizing trading strategies.

What is the Normal SABR Model?

The Normal SABR model is a mathematical formula used in finance to estimate the volatility of an asset over time. It is particularly useful in pricing and valuing options, which are financial derivatives that give the holder the right, but not the obligation, to buy or sell an underlying asset at a specified price (the strike price) on or before a specific date (the expiration date).

The name “SABR” is an acronym for “Stochastic Alpha, Beta, Rho,” which refers to the parameters used in the model. The model was originally introduced by Hagan et al. in 2002 as an extension of the popular Black-Scholes-Merton model, which assumes that asset price movements are normally distributed. The Normal SABR model relaxes this assumption and allows for skewness and kurtosis in the distribution of asset returns.

The Normal SABR model is based on the concept of stochastic volatility, which posits that asset price fluctuations are driven by the volatility of the underlying asset itself. In other words, the volatility is not constant, but rather varies over time. This is in contrast to the Black-Scholes-Merton model, which assumes constant volatility.

The Normal SABR model is often used in the pricing and risk management of exotic options, such as barrier options and digital options, which have features that make them more complex than plain vanilla options. The model allows for a more accurate estimation of the true volatility of the underlying asset, taking into account the skewness and kurtosis of the distribution of asset returns.

The Normal SABR model is widely used in the financial industry. It has become an industry standard for pricing and valuing options and is implemented in many risk management systems and trading platforms. Understanding how the Normal SABR model works is essential for professionals working in quantitative finance, options trading, and risk management.

Read Also: Complete Guide to Free Trading: Tips and Strategies for Successful Trading

Benefits of the Normal SABR model:
Accurate estimation of volatility for pricing and valuing options
Ability to take into account skewness and kurtosis in distribution of asset returns
Widely used in financial industry
Standard for pricing and risk management of exotic options

Key Features of the Normal SABR Model

The Normal SABR model is a popular stochastic volatility model used in option pricing. It is an extension of the original SABR model, which was developed by Hagan et al. in 2002. The Normal SABR model allows for more flexible modeling of the volatility by assuming a normal distribution for the instantaneous volatility instead of a log-normal distribution.

Here are some key features of the Normal SABR model:

Volatility Skew: The Normal SABR model is able to capture the volatility skew commonly observed in option markets. The model allows for a non-linear relationship between the strike price and implied volatility, which is consistent with market observations.
Volatility Structure: The model is able to capture the dynamics of the volatility structure, including the mean reversion and the correlation between the volatility and the underlying asset price.
Finite Moments: Unlike some other stochastic volatility models, the Normal SABR model ensures that the moments of the asset price and volatility are finite, which is important for option pricing and risk management.
Parameter Calibration: The model includes four key parameters that need to be calibrated to market data: the initial volatility, the correlation between the volatility and the underlying asset price, the mean reversion speed of the volatility, and the volatility of the volatility. Proper calibration of these parameters is crucial for accurate option pricing.
Efficient Numerical Methods: Various numerical methods have been developed for efficiently pricing options under the Normal SABR model. These methods allow for fast and accurate option pricing, making the model practical for real-world applications.

The Normal SABR model is widely used in the financial industry for pricing and risk management of options. Its ability to capture the volatility skew and volatility structure, along with its efficient numerical methods, makes it a valuable tool for option traders and risk managers.

Read Also: 5 Tips to Avoid Mistakes in Option Trading and Maximize Profits

FAQ:

What is the SABR model?

The SABR model is a mathematical model used to price derivative products in finance, particularly interest rate options. It stands for Stochastic Alpha, Beta, Rho, and is named after the four parameters used in the model.

How does the SABR model work?

The SABR model assumes that the underlying asset follows a geometric Brownian motion and the volatility of the asset follows a stochastic process. The model uses four parameters - alpha, beta, rho, and volvol - to describe the dynamics of the asset price and volatility.

What are the main advantages of the SABR model?

The main advantages of the SABR model are its ability to handle skew and smile in option prices, its flexibility in capturing market dynamics, and its ability to accurately price and hedge exotic options.

What are some of the limitations of the SABR model?

Some limitations of the SABR model include its assumption of constant parameters, the need for calibration to market data, and its sensitivity to extreme market conditions. Additionally, the model may not accurately capture dynamics in illiquid markets.

How is the SABR model calibrated to market data?

The SABR model is typically calibrated to market data using optimization techniques, such as minimizing the difference between observed and modeled option prices. This calibration process involves finding the values of the four SABR parameters that best fit the market data.

What is the SABR model?

The SABR model is a financial model used to price derivatives, particularly interest rate options.

How does the SABR model work?

The SABR model uses a stochastic volatility approach to account for the volatility smile, an observed feature in the options market where implied volatility is not constant across different strikes.