Pros and Cons of Using the Average of Averages

Advantages and Disadvantages of the Average of Averages

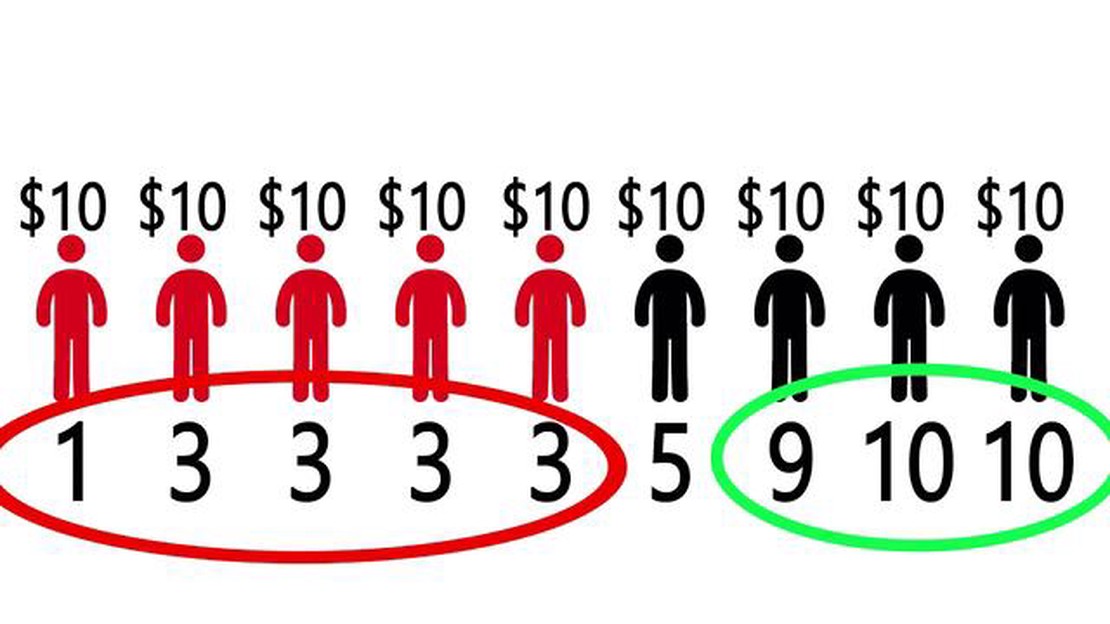
The concept of using the average of averages is a common practice in statistical analysis, but it comes with its pros and cons. This method involves calculating the average of multiple averages, which can be useful in certain situations, but it also has its limitations.

Table Of Contents

One of the advantages of using the average of averages is that it can help in dealing with data that is divided into subgroups. By calculating the average of each subgroup and then taking the average of those averages, you can get a more comprehensive understanding of the overall data. This can be especially useful when comparing different groups or analyzing trends over time.

However, it is important to keep in mind that using the average of averages can sometimes mask important variations within subgroups. If the data within each subgroup is very different from each other, the overall average may not accurately represent the true picture. In such cases, it would be more appropriate to use other statistical measures or analyze the data on a subgroup level.

Another advantage of using the average of averages is that it can simplify complex data sets. By condensing the data into a single average, it becomes easier to interpret and communicate the findings to a broader audience. This can be particularly useful in presenting data in a clear and concise manner.

On the other hand, the average of averages can also introduce bias into the analysis. This method gives equal weight to each subgroup, regardless of their size. As a result, smaller subgroups may have a disproportionate influence on the overall average. This can lead to misleading interpretations and inaccurate conclusions.

In conclusion, the decision to use the average of averages should be made carefully, taking into consideration the specific context and goals of the analysis. While it can provide a simplified and comprehensive overview of the data, it may also overlook important variations within subgroups and introduce bias. Understanding the pros and cons of this method is crucial for making informed and accurate statistical interpretations.

Pros of Using the Average of Averages

Using the average of averages has several advantages in certain situations:


1. Straightforward Calculation	Calculating the average of averages is a simple and straightforward process. It involves taking the average of individual averages, which can be easily done using basic mathematical operations.
2. Accounting for Variations	By calculating the average of averages, you can account for variations in data. This method considers all the individual averages, giving equal weight to each of them. It provides a balanced view of the overall data set.
3. Highlighting Trends	The average of averages can help highlight trends in the data. By comparing the average values over different periods or groups, you can identify patterns or changes that might not be apparent when looking at the data as a whole.
4. Simplifying Complex Data	In cases where you have complex data with multiple variables or subgroups, using the average of averages can simplify the analysis. It provides a summary metric that is easier to interpret and communicate.
5. Handling Missing Data	If you have missing data points in your dataset, using the average of averages can help mitigate the impact of those missing values. It ensures that the overall average takes into account all available data, even if some individual averages are missing.

Overall, the average of averages can be a useful tool for analyzing and interpreting data, especially in situations where you want to account for variations, identify trends, simplify complex data, or handle missing values.

Enhanced Precision

One of the key benefits of using the average of averages is the enhanced precision it provides. By calculating the average of multiple averages, we are able to obtain a more accurate and representative measure of the overall data set.

When we calculate the average of averages, we are effectively giving each average a weight based on the number of data points it represents. This means that larger averages, which are based on more data points, will have a greater influence on the overall result.

By taking into account the number of data points in each average, we can account for any discrepancies or outliers that may exist within smaller subsets of the data. This can help to reduce the impact of any anomalies and give a more accurate representation of the underlying data pattern.

Furthermore, by using the average of averages, we can also mitigate the issues that may arise from extreme values. Extreme values in a single average can skew the overall result, making it less representative of the entire data set. By combining multiple averages, we can spread the impact of these extreme values and obtain a more stable measure of the data.

Read Also: Understanding the Moving Average Gradient and Its Importance in Data Analysis

In conclusion, the average of averages provides enhanced precision compared to calculating a single average. It takes into account the number of data points in each average and helps to reduce the influence of anomalies and extreme values. This makes it a valuable tool for obtaining a more accurate representation of the underlying data set.

Easy to Understand and Interpret

One of the advantages of using the average of averages is that it is relatively easy to understand and interpret. The concept of averaging is well-known and widely used, making it familiar to most people.

Read Also: Discover the Truth: Are Forex Signals Real or Just a Scam?

When using the average of averages, you simply calculate the average value of each group and then take the average of those averages. This provides a single value that represents the overall average of the data.

This simplicity makes it easy for individuals to grasp the meaning of the average of averages and interpret it in a meaningful way. It is a straightforward method that does not require complex calculations or advanced statistical knowledge.

Furthermore, the average of averages can be easily communicated and explained to others. As a result, it can be helpful in presenting data and findings to a non-technical audience or stakeholders who may not be familiar with more complex statistical measures.

In addition, the ease of understanding and interpretation can make the average of averages a useful tool for making comparisons. By calculating the average of averages for different groups or time periods, you can easily compare the overall averages and identify any noticeable differences.

Group	Average
Group 1	80
Group 2	85
Group 3	75

In the example table above, you can see the average values for three different groups. By taking the average of these averages (80 + 85 + 75) / 3 = 80, you can determine the overall average for the data set.

Overall, the easy understandability and interpretability of the average of averages make it a practical and accessible method for analyzing and presenting data.

FAQ:

What is the average of averages method?

The average of averages method is a statistical technique for calculating an overall average by first finding the averages of different subgroups and then taking the average of those averages.

What are the advantages of using the average of averages?

There are several advantages of using the average of averages method. Firstly, it can provide a general overview of the data by condensing multiple averages into a single value. Secondly, it is a simple and straightforward method that can be easily understood and implemented. Finally, it can be useful in situations where the subgroups have equal importance and need to be weighted equally in the final average.

What are the disadvantages of using the average of averages?

While the average of averages method has its advantages, it also has some potential disadvantages. One drawback is that it may not accurately represent the overall data, as it is based on the averages of subgroups rather than the raw data points. Additionally, the method assumes that all subgroups have equal importance, which may not always be the case. Finally, if the subgroups have different sizes, the resulting average may be skewed.

Are there any alternative methods to the average of averages?

Yes, there are alternative methods to the average of averages. Some common alternatives include weighted averages, where the subgroups are assigned different weights based on their importance, and median averages, where the middle value of the data set is used instead of the mean. These methods can provide a more accurate representation of the data in certain situations.