Why Use Weighted Average vs Average: Understanding the Difference

Why choose weighted average over average?

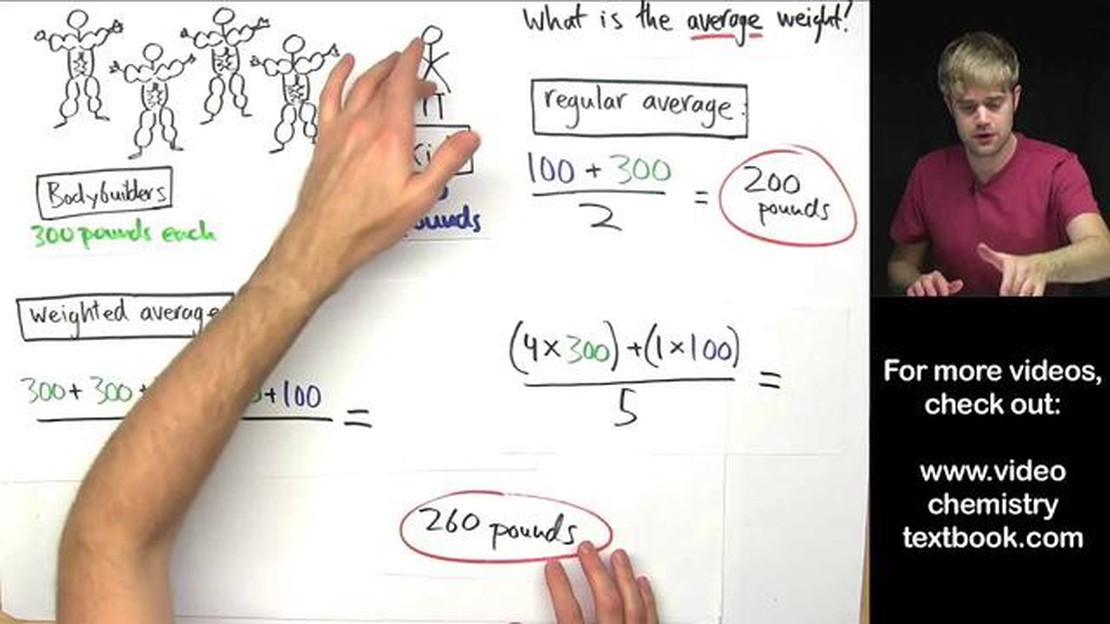
When it comes to calculating averages, there are different methods to consider, such as the weighted average and the simple average. While both methods aim to provide a representative value, they differ in their approach and application. Understanding the difference between these two methods is crucial for making informed decisions in various fields, including finance, statistics, and data analysis.

Table Of Contents

The simple average, also known as the arithmetic mean, is perhaps the most commonly used method for calculating averages. It involves summing up all the values in a dataset and dividing the total by the number of values. The result represents the “typical” value in the dataset. This method works well when all values are equally important and should be given equal weight in the calculation.

On the other hand, the weighted average takes into account the importance or significance of each value in the dataset. This method assigns weights to each value based on predetermined factors or criteria. The weighted average is especially useful when different values contribute differently to the overall outcome. For example, in financial analysis, stock prices with a higher market capitalization may have a greater impact on the overall index than stocks with lower market capitalization.

In conclusion, whether to use the weighted average or the simple average depends on the nature of the data and the purpose of the calculation. The simple average provides a quick and easy way to calculate the average for straightforward datasets, while the weighted average allows for a more nuanced representation when certain values carry more significance. Understanding the difference between these methods will help ensure accurate and meaningful calculations in various domains.

Why Use Weighted Average vs Average

When analyzing data or calculating the average of a set of numbers, it is important to consider the significance or weight of each value. This is where the concept of weighted average comes into play.

By using a weighted average, you can assign different weights to each value based on their importance or contribution to the overall result. This allows for a more accurate representation of the data.

A weighted average is particularly useful when dealing with situations where certain values have more influence or significance than others. For example, in financial analysis, stock prices with higher market capitalizations may carry more weight in calculating the average stock price.

In contrast, a simple average treats all values equally and does not take into account any variations in importance. While this can work well in certain scenarios, it may not accurately reflect the underlying data in other cases.

Weighted averages are commonly used in various fields, including finance, statistics, economics, and decision-making processes. They provide a way to incorporate varying degrees of importance or significance into calculations, resulting in more reliable and meaningful results.

Overall, using a weighted average vs a simple average allows for a more comprehensive analysis of data and provides a more accurate representation of the underlying information. It enhances decision-making processes and helps draw more informed conclusions.

Read Also: Understanding the Meaning of Clearing in Trading - All You Need to Know

Weighted Average	Average
Takes into account the significance or weight of each value	Treats all values equally
Provides a more accurate representation of the data	May not accurately reflect the underlying data in certain cases
Used in fields like finance, statistics, economics	Used in simple calculations
Enhances decision-making processes	May not provide comprehensive analysis

Understanding the Difference

Understanding the difference between weighted average and average is essential for accurately assessing data. The two concepts may seem similar at first, but they have distinct purposes and functions.

When calculating an average, all data points are given equal weight. This means that every value contributes equally to the overall result. While this is useful in some cases, it can also be misleading when dealing with data sets that have outliers or extreme values. In such cases, using a weighted average can provide a more accurate representation of the data.

Weighted average takes into account the significance or importance of each data point when calculating the average. This is done by assigning weights to each value based on certain criteria. These criteria could be the volume or proportion of a specific data point relative to the overall data set, or external factors that affect the significance of certain values. By incorporating weights, a weighted average can give more weight or importance to certain values, resulting in a more accurate representation of the data.

Weighted average is particularly useful in situations where some data points have a larger impact on the overall result than others. For example, when calculating a grade point average (GPA), the grade received in each class is often weighted based on the number of credit hours for that class. This means that a higher weight is given to classes with more credit hours, reflecting their larger impact on the overall GPA.

In contrast, a regular average treats all data points equally, regardless of their significance. This can lead to skewed results if outliers or extreme values are present in the data set. By giving equal weight to all values, the regular average may not accurately represent the central tendency of the data.

Read Also: What time does the market close in Malaysia? Find out the closing hours here!

In summary, the main difference between weighted average and average lies in the consideration of the significance or importance of each data point. While an average treats all values equally, a weighted average takes into account the weights assigned to each value. This makes the weighted average a more powerful tool for analyzing data sets with varying degrees of importance or significance among the data points.

Weighted Average	Average
Takes into account the significance or importance of each data point	Treats all data points equally without considering their significance
Uses weights assigned to each value	Assigns equal weight to all values
Provides a more accurate representation of the data	May lead to skewed results if outliers or extreme values are present

FAQ:

What is the difference between weighted average and average?

Weighted average and average are both measures of central tendency, but they calculate the value in different ways. Average is a simple arithmetic mean, which is calculated by summing up all the values in a dataset and dividing by the number of values. Weighted average, on the other hand, takes into account the importance or weight of each value, and calculates the average by multiplying each value by its respective weight and then dividing by the sum of the weights.

When should I use weighted average instead of average?

You should use weighted average instead of average when the values in your dataset have different importance or weight. For example, in a grade calculation where each assignment is worth a different percentage of the total grade, using weighted average will give a more accurate representation of the overall performance. In situations where each value has equal weight, average is sufficient.

How do you calculate a weighted average?

To calculate a weighted average, you multiply each value by its weight, then sum up all the products. Finally, divide the sum by the sum of the weights. The formula for weighted average is: weighted average = (value1 * weight1 + value2 * weight2 + … + valueN * weightN) / (weight1 + weight2 + … + weightN)

What are some real-life examples where weighted average is used?

Weighted average is used in various real-life examples, such as calculating GPA (Grade Point Average) where each course is worth a certain number of credits. It is also used in financial calculations, like calculating portfolio returns where each investment has a different weight. Additionally, weighted average is used in market research to calculate indices or satisfaction scores, where different attributes are given different weights based on their importance.

Are there any disadvantages of using weighted average?

One potential disadvantage of using weighted average is that it requires assigning weights to each value, which can be subjective and may introduce bias. In cases where the weights are not accurately determined, the weighted average may not accurately represent the true central tendency. Additionally, calculating weighted average can be more time-consuming and complex than calculating simple average.