Discover the Most Famous Fractal and its Fascinating Properties

Exploring the Most Famous Fractal: Discovering the Beauty of the Mandelbrot Set

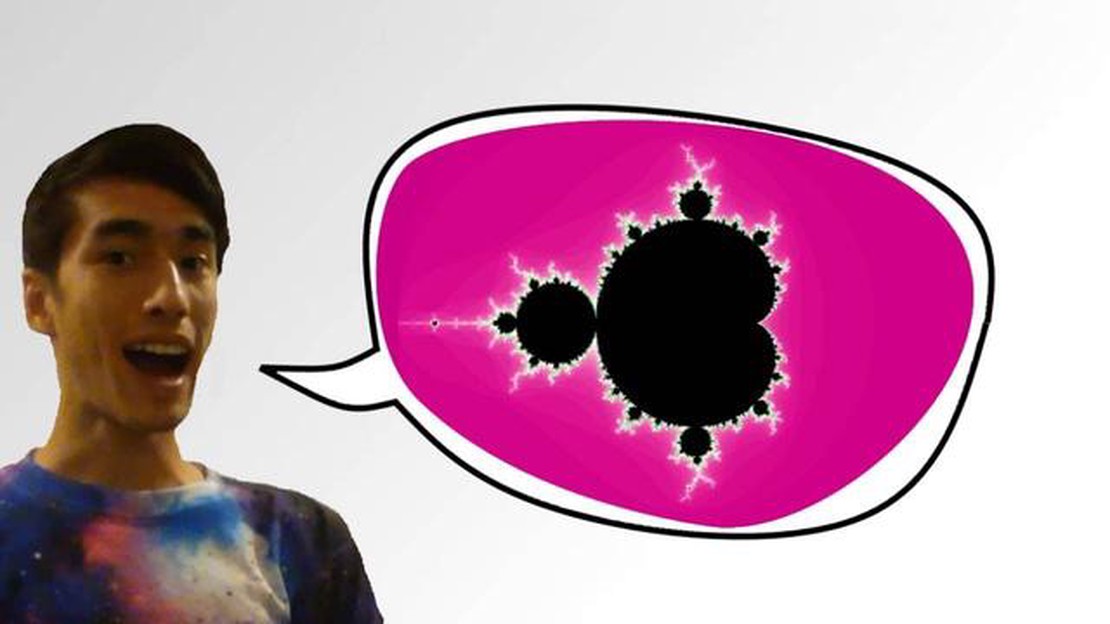
Fractals are complex mathematical shapes that exhibit self-similarity and infinite detail. These mesmerizing patterns can be found in nature, art, and even in the realm of mathematics itself. One of the most famous fractals is the Mandelbrot set.

The Mandelbrot set, discovered by mathematician Benoit Mandelbrot in 1979, is a fascinating and intricate fractal that has captured the imaginations of both mathematicians and the general public. The set is defined in the complex plane and is generated by iteratively applying a simple function to a given starting point.

Table Of Contents

What makes the Mandelbrot set so interesting is its infinite complexity and endless variation. As you zoom in on different areas of the set, intricate patterns and shapes emerge, revealing finer and more intricate details. The boundary of the set itself is a fractal, with intricate filaments and spirals that stretch to infinity.

Not only is the Mandelbrot set visually stunning, but it also has deep mathematical and philosophical implications. The set has connections to complex dynamics, chaotic behavior, and the nature of infinity itself. The study of the Mandelbrot set has led to numerous discoveries in mathematics and has opened up new avenues of inquiry in the field of fractal geometry.

The Most Famous Fractal

The fractal known as the Mandelbrot set is perhaps the most famous and well-known fractal in the world. Named after the mathematician Benoit Mandelbrot, who discovered it in 1978, the Mandelbrot set has captivated mathematicians, artists, and enthusiasts alike with its intricate and infinitely complex structure.

The Mandelbrot set is generated by a simple mathematical equation that involves complex numbers. Starting with a chosen complex number, the equation is iteratively applied to create a sequence of numbers. If this sequence remains bounded, the starting complex number is a part of the Mandelbrot set, and if it diverges to infinity, the complex number is not part of the set.

One of the most fascinating properties of the Mandelbrot set is its self-similarity. Regardless of the level of zoom, the overall shape of the set remains the same. As you zoom in, intricate patterns and details emerge, revealing an incredible level of complexity. The Mandelbrot set also exhibits both infinite complexity and infinite simplicity, with an infinite number of intricate details and structures, yet its essence can be represented by a simple equation.

Due to its aesthetic appeal and visually stunning patterns, the Mandelbrot set has become synonymous with fractals and has inspired numerous artists and musicians. Its intricate and infinitely repeating patterns have found applications in various fields, including computer graphics, physics, and data visualization.

From its initial discovery, the Mandelbrot set has become an iconic representation of the beauty and complexity of mathematics. Its visually appealing patterns and mathematical properties continue to fascinate and inspire mathematicians and enthusiasts, making it the most famous fractal in the world.

The History and Origins

The most famous fractal, known as the Mandelbrot set, was discovered by mathematician Benoit Mandelbrot in the 1970s. Mandelbrot was born in Poland in 1924 and moved to France at a young age. He studied mathematics at the prestigious Ecole Polytechnique in Paris before obtaining a Ph.D. from the University of Paris.

Mandelbrot became interested in the beauty and complexity of mathematical patterns early on in his career. He coined the term “fractal” to describe these intricate structures that exhibit self-similarity at all levels of magnification. The Mandelbrot set, which is named after its discoverer, is perhaps the most famous manifestation of fractal geometry.

The complex numbers and the concept of iteration play a central role in the creation and exploration of the Mandelbrot set. The set itself is defined as the set of complex numbers C for which the sequence of numbers defined by the iteration function Z(n+1) = Z(n)^2 + C remains bounded. The complex plane is then divided into two regions: the black region inside the set and the colorful region outside the set.

The Mandelbrot set gained widespread attention in the 1980s when computer graphics made it possible to visualize the intricate details of the fractal. The stunning images produced by iterating the equation for different values of C captivated both mathematicians and non-mathematicians alike.

Today, the Mandelbrot set continues to be a source of fascination and study. It has been explored in depth by mathematicians, computer scientists, and artists. Its intricate patterns and infinite complexity continue to inspire and captivate researchers and enthusiasts around the world.

The Intricate Patterns

The most famous fractal, known as the Mandelbrot set, is renowned for its intricate and complex patterns. When zoomed in, the Mandelbrot set reveals a never-ending sequence of self-similar patterns, revealing hidden details with each level of magnification.

Read Also: Can you short synthetic shares? Explained by experts

The patterns of the Mandelbrot set are created using a simple mathematical formula, which involves iterating the complex numbers and determining whether they are within a specific bounded region. The points that remain bounded form the intricate patterns of the set, while the points that escape to infinity create the background area.

One of the fascinating properties of the Mandelbrot set is its infinite complexity. No matter how close you zoom in, there are always new patterns and details to explore. This infinite complexity is a result of the set’s self-similarity at different scales, meaning that similar patterns are repeated at all levels of magnification.

Read Also: Choosing the Best Broker in Australia: A Comprehensive Guide

In addition to its complexity, the Mandelbrot set also exhibits fractal symmetry. This means that its fascinating patterns are replicated in smaller sections of the set, leading to a never-ending repetition of shapes and structures. Fractal symmetry adds to the aesthetic appeal of the set and makes it visually captivating.

Furthermore, the intricate patterns of the Mandelbrot set have captured the attention of mathematicians, artists, and enthusiasts alike. The set has inspired various forms of art and creative expressions, as its mesmerizing patterns evoke a sense of awe and wonder.

Overall, the Mandelbrot set’s intricate patterns, infinite complexity, fractal symmetry, and aesthetic appeal have secured its place as the most famous fractal and continue to fascinate those who explore its mesmerizing beauty.

The Mathematical Properties

Fractals are complex geometric shapes that exhibit self-similarity on different scales. The most famous fractal, known as the Mandelbrot Set, has numerous captivating mathematical properties.

One of the most fascinating properties of the Mandelbrot Set is its infinite complexity. No matter how much you zoom into the fractal, there will always be more intricate details to discover. This infinite complexity arises from the iterative equation used to generate the Mandelbrot Set.

Another notable property of the Mandelbrot Set is its boundary, also known as the complex coastline. The boundary of the set is highly detailed and exhibits a fractal structure. As you zoom closer to the boundary, you will encounter intricate patterns and intricate shapes that repeat on different scales.

The Mandelbrot Set also exhibits self-similarity. This means that zooming into one part of the fractal will reveal smaller copies of the entire set. You can continue zooming infinitely and still find the same structure repeating, albeit at a smaller scale. This property is a fundamental characteristic of fractals.

Furthermore, the Mandelbrot Set is connected to complex numbers and the concept of iteration in mathematics. The equation used to generate the set involves repeatedly feeding complex numbers back into the equation. The set’s structure emerges from the behavior of these iterated complex numbers.

The Mandelbrot Set is also known for its infinitely intricate boundary, known as the complex coastline. This boundary is not smooth but exhibits intricate patterns and shapes that repeat on different scales. This complexity is a result of the iterative nature of the equation used to generate the set.

In conclusion, the Mandelbrot Set possesses a multitude of captivating mathematical properties. Its infinite complexity, self-similarity, and intricate boundary make it a fascinating subject of study for mathematicians and enthusiasts alike.

FAQ:

What is a fractal?

A fractal is a complex mathematical pattern that repeats itself infinitely at different scales.

What is the most famous fractal?

The most famous fractal is the Mandelbrot Set.

What are some properties of the Mandelbrot Set?

The Mandelbrot Set is infinitely complex, self-replicating, and has intricate patterns within patterns.

How is the Mandelbrot Set generated?

The Mandelbrot Set is generated by iterating a simple equation for each point on the complex plane and determining if the result tends towards infinity or stays within a certain range.